Monochromatic Aberrations

 

What are monochromatic aberrations?

Monochromatic aberrations are aberrations that occur in quasimonochromatic light.  These aberrations do not consider the effect the frequency of light has on its propagation through a system. (Real light is never monochromatic - it always is made of a band of frequencies.  However, when this band is narrow, the light is considered to be quasimonochromatic.)  These are aberrations typically related to the paraxial approximation, which assumes that all light is entering the system nearly parallel to each other and perpendicular to the lens.  In real systems, this is not the case. 

            There are five primary monochromatic aberrations (also called Seidel aberrations), which are divided into two subgroups:

            The first subgroup includes aberrations that deteriorate the image:

I.                    Spherical aberration

II.                 Coma

III.               Astigmatism

The second subgroup includes aberrations that deform the image:

IV.              Petzval field curvature

V.                 Distortion

 

How are monochromatic aberrations described mathematically?

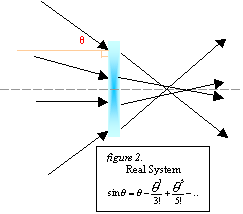
In an ideal system (figure 1), we use the expansion .  To consider the monochromatic aberrations of a real system (figure 2), we need to keep another term in the expansion making:

            Using this approximation to look at an axial ray through point Q (figure 3), we find the aberration (a(Q))to be:

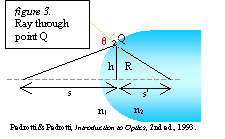
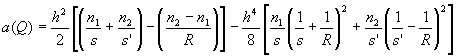
The first term in this expression, , is the expression found using first order theory.  It is equal to zero by Fermat’s Principle.

The expression, therefore, reduces to:

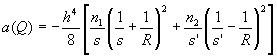
            All of the terms except are constant to the specific system, meaning that they can be considered a single constant (C), giving us .

            If we consider an off axis aberration, using third-order theory (as we just did), we find

            There are five terms in this expression which correspond to each of the five main monochromatic aberrations:

                                                                               Spherical Aberration

                                                                   Coma

                                                               Astigmatism

                                                                          Curvature of Field

                                                                   Distortion

 

            where h' is the distance from axial imaging, r is the aperture size, and θ is the symmetry around the axis.

 

Pedrotti, Frank, & Leno Pedrotti, Introduction to Optics, 2nd edition, New Jersey: Prentice Hall, 1993.